
Mathematics is the most abstract language of system models
Mathematical formulas represent sequences of phenomena which follow the same principles.
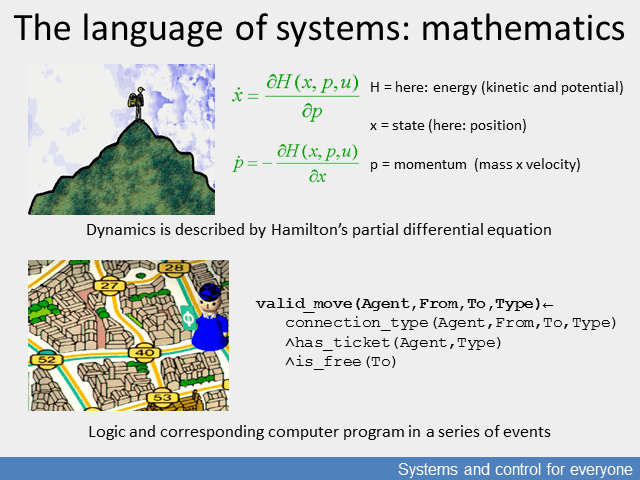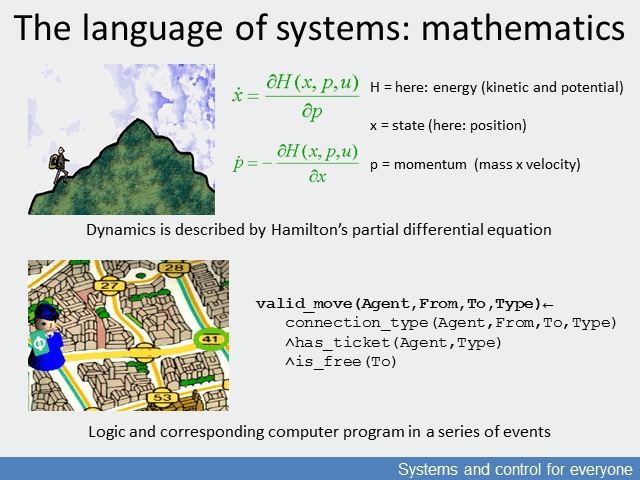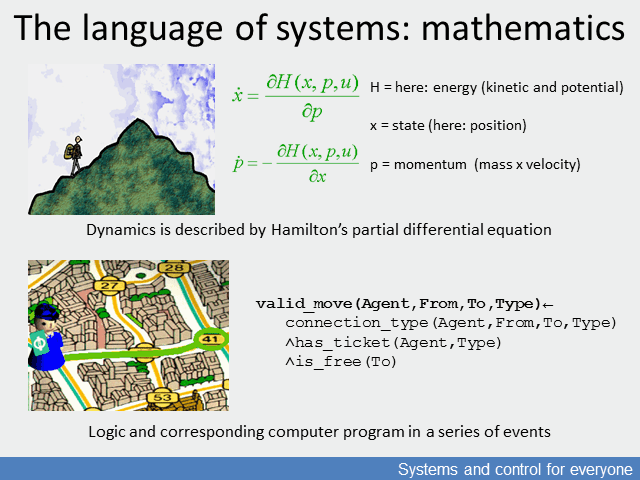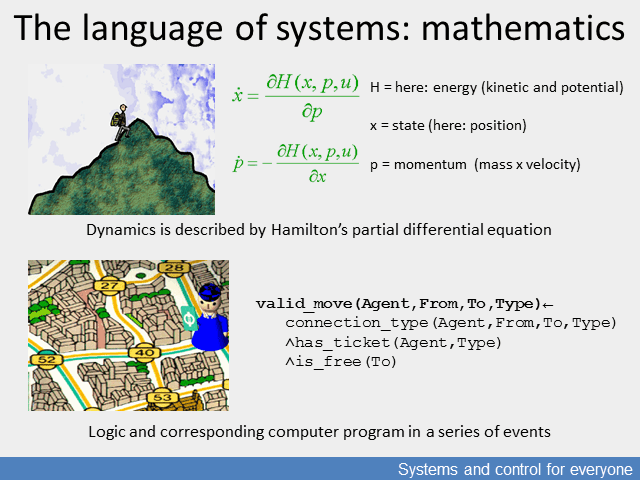
Let’s go back for a second to the pictures about homomorphism and homology. Examples of the general motion of fluids (waterfall), the physics of upthrust (aerodynamic force), etc. can be described by similar mathematical equations. The most general Hamiltonian interpretation of motion characterizes the behaviour of dynamical processes. The latter interpretation will appear here several times as this is the most general definition of dynamics and dynamic equilibriums, used as a basic physical formula both in the control of systems, in quantum physics and other phenomena.
All electromagnetic phenomena are summarised by Maxwell’s four equations. The basic concepts of thermodynamics and information theory are described by the entropy equation.
The succession of systems, and system parts have a similarly general mathematical description, which use the formalism of mathematical logic.
The most abstract model of thinking about systems is the mathematical representation which became a highly useful practical tool with the emergence of computer science. The development of the mathematical model is also the result of a long evolution which started with the listing of farm animals and objects through the comparison of travel distances to the abstract levels of modern mathematics.
There are primarily differential equations in the mathematical representation, and the solution of design and scheduling tasks of systems in the time domain. The Taylor and Fourier series and their various transformations, and in control topics the Laplace transforms are used for analyses by frequency components. The behaviour in the time domain is visualised in the Cartesian coordinate system, the frequency aspect, behaviour in the frequency domain is visualised in the vector space of complex variables. For further textual and visual information about these concepts and methods the search bar can be used.