
Is there an answer? And if so, how definitive is it?
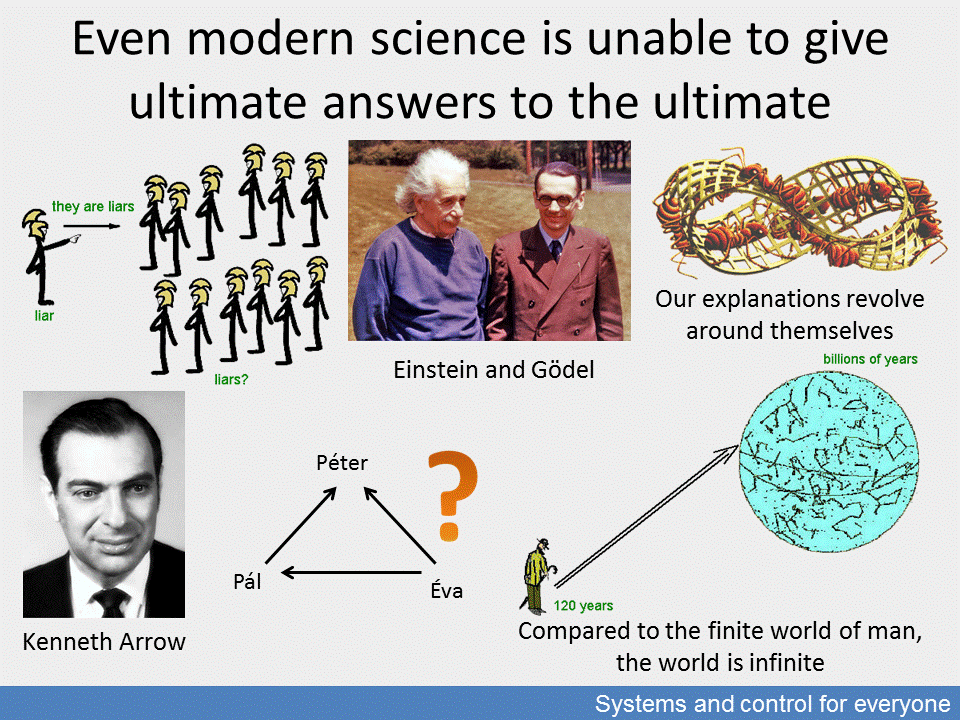
The answer is simple: there is no definitive answer. This is what follows from our epistemological remarks above but also from Gödel’s theorems of mathematical logic which states more or less the following: in a closed logical system there is always one thesis which cannot be proved within the framework of that system. The philosophical and practical generalisation follows naturally from this: thinking corresponds to the concept of the closed system, as it is closed into our great empirical world and time constraints.
Gödel refers to the classical Cretan liar paradox in which a Cretan states that “all Cretans are liars”, that is within the closed system of Cretans the truth of this sentence is not decidable. Humanity is Cretan in its entirety and in respect of its history as well!
Another similar impossibility proof concerns voting and the reconciliation of values. Arrow’s theorem states that the choice of values in case multiple participants cannot be ranked in reasonable order, i.e. there is no absolute decisive system if multiple values are involved. This becomes even more plastic if values and aspects change over time.
Thus, both in terms of our knowledge about the world and the relationships between people, that is in terms of our external and internal systems approach, no final and absolute truths can be proclaimed.
Cognition has various layers. In the course of cognition we determine the purpose of the examination, highlight what is important and ignore what is not, and specify the model’s scope of validity.