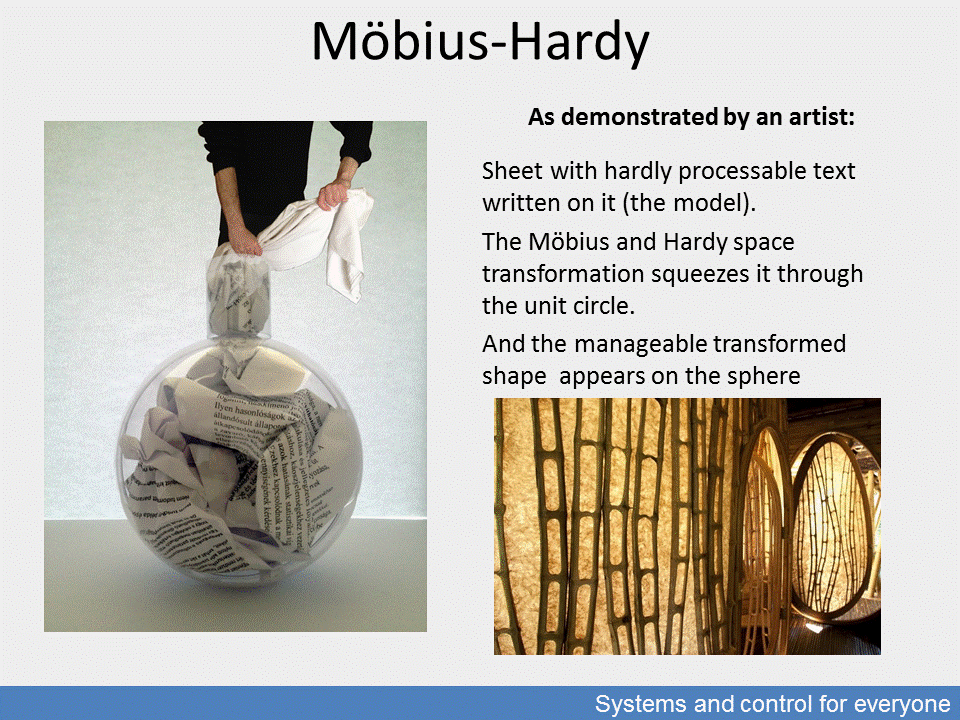

Hardy spaces
Hardy spaces are system spaces which are characterised by measures and which are separated by suprema, i.e. upper limits in the spaces describing the processes. This space representation can take place on the complex plain or—by transformation—within and on the boundaries of a unit circle as the chosen spaces include both representation and calculation by time and by frequency.
In practice, the most often used version is the one transformed into a circle which solves the problem of managing endlessly running processes. In the Hardy space the stability and quality indicators of a process is characterised by the measures derived from the integral of the processes.
This cognitive, mathematical abstractness makes it more difficult to effect transitions from more practical methods that work with simpler models, still it has become the general methodology for dealing with difficult problems in the past three decades.
It is mentioned here because it is important to know about the trends influencing the application of system theory. More details of the topic will be presented in Chapter XI which is a more technical account of the problem and therefore relies more on the reader’s mathematical background knowledge.