
The Möbius transformation
The video below is an excellent visualisation of the transformation.
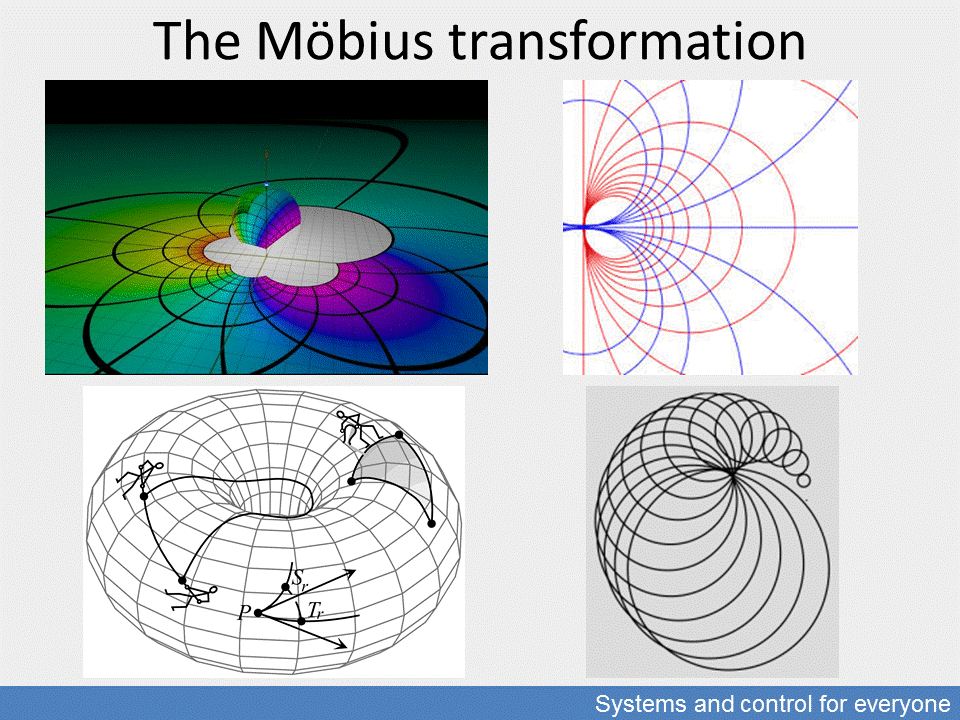
The Möbius transformation rotates and proportionally (with a linear measure) distorts the shapes of the Euclidean space. In our applications it transforms spaces extending to infinity and the processes in them into the inside of a circle. This is usually a unit circle and therefore represents the inversion created by the feedback of the process.
The transformation is applied to the complex plain where the states of the process are depicted with vectors. The length of the vector is the amplification or decrease caused by the process, and its phase angle represents the time shift between the input and the output of the process.
The Möbius transformation can be performed on a group of processes which can be described with polynomials, i.e. holomorphic functions that can be differentiated everywhere in the given section, therefore it can be used in a large variety of continuous processes in practice.
With its shrinking, expanding, rotating effect, the Möbius transformation is part of all image processing methods which support the examination of the details, relations and diversity of a problem, while conserving the examined properties with the strictness of group properties and authentically ensuring that the images remain back-transformable.
It is equally important in the examination of phenomena abstracted from visual representations and described by mathematical-computational tools, as well as in the problems of stability and system quality. In modern systems theory, such problems are primarily examined in Hardy spaces which are also concepts created through Möbius transformation.